Table of contents
Numbers are more than just objects or quantities that we can see and touch. They are ideas that go beyond the physical world. They represent quantities and magnitudes and serve as a fundamental part of mathematics. Take the number "3" as an example. It can represent three apples, three kilometers, or even three minutes. Regardless of what it represents, the number "3" holds a consistent value. This consistency allows us to compare different quantities, perform calculations, and establish relationships between numbers.

Numbers provide a universal language for communication and analysis, allowing us to make comparisons, perform calculations, and establish relationships across various contexts.
Related Topics
| Natural Numbers | Whole Numbers |
| Prime Numbers | Roman Numerals |
| Integers | Fractions |
| Decimal Numbers | Rational Numbers |
Complex Numbers | Fibonacci Numbers |
Irrational Numbers | Real Numbers |
Digits and Numerals
Imagine numerals as special signs that represent different numbers. Just like how we have letters to write words, we use numerals to express numbers. For example, the numeral "7" represents the number seven. So, numerals are like codes that tell us the value of the number.

Now, let's talk about digits. Digits are like tiny puzzle pieces that help create numerals. We have ten special symbols called digits: 0, 1, 2, 3, 4, 5, 6, 7, 8, and 9. These digits are the building blocks of numerals.
For instance, the numeral 64 is formed by combining two digits: "6" and "4." Even a single digit, like "2," can be a numeral by itself, representing the number two.
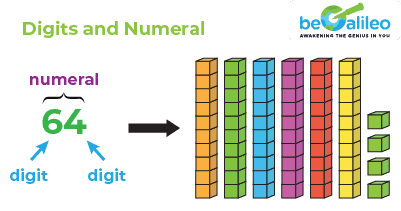
So, think of numerals as secret codes for numbers, and digits as the unique puzzle pieces used to create these codes. Understanding numerals and digits allows us to read and write numbers easily, which is important for various activities in our everyday life and mathematics.
Numeration Systems
In mathematics, we use various writing systems, known as numeration systems, to represent numbers. The one we commonly encounter employs digits from 0 to 9, and it is called the Hindu-Arabic system. This system allows us to write all kinds of numbers using these digits.
Additionally, you might have come across Roman Numerals like VI and X, which are part of the Roman Numeration System. Roman Numerals have a different set of symbols and rules for expressing numbers.
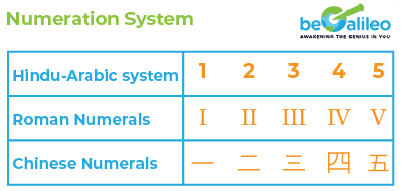
Each numeration system has its unique way of representing numbers, making math fascinating and diverse.
History of Numbers
The history of numbers dates back thousands of years and is closely intertwined with the development of human civilization. Numbers have played a crucial role in various aspects of human life, including trade, agriculture, architecture, astronomy, and more. Let's take a brief journey through the history of numbers
The earliest evidence of numerical systems can be traced back to ancient civilizations such as the Sumerians, Egyptians, and Indus Valley Civilization. These civilizations developed their own numeral systems to represent quantities and perform calculations.
The Sumerians used a base-60 system, which led to the creation of the concept of minutes and seconds in measuring time. The Egyptians employed a decimal system, using hieroglyphs to represent numbers.
One of the most significant developments in the history of numbers came from the ancient Indian mathematicians. Around the 5th century BCE, the Indians introduced the concept of zero and the decimal place-value system. This revolutionary idea of positional notation made arithmetic operations more efficient and laid the foundation for modern mathematics. It eventually spread to other parts of the world, including the Arab world.
During the Islamic Golden Age, scholars such as Al-Khwarizmi made significant contributions to mathematics. Al-Khwarizmi's book "Kitab al-Jabr wa al-Muqabala" introduced algebra and algorithms, which further advanced the understanding and use of numbers.
In Europe, the adoption of Arabic numerals, which included the digit zero, gained prominence during the Middle Ages. It revolutionized mathematical computations and made complex calculations more accessible. The Italian mathematician Fibonacci introduced the Hindu-Arabic numeral system to Europe in the 13th century, further popularizing its use.
The Renaissance period witnessed significant advancements in mathematics, with notable mathematicians like Leonardo da Vinci and Galileo Galilei contributing to the understanding of numbers and their applications. The Scientific Revolution of the 17th century, led by scientists such as Sir Isaac Newton and Gottfried Wilhelm Leibniz, brought about further developments in mathematics, including the invention of Calculus.
In modern times, the field of mathematics has witnessed remarkable progress, with the exploration of complex numbers, set theory, number theory, and the discovery of irrational and transcendental numbers. These developments have greatly expanded our understanding of numbers and their relationships.
Counting Numbers
Counting Numbers also known as natural numbers, are the numbers we use to count or enumerate objects. They start from 1 and continue indefinitely. The set of counting numbers is denoted as N = {1, 2, 3, 4, 5, ...}.
Example: Counting numbers from 1 to 10 are 1, 2, 3, 4, 5, 6, 7, 8, 9, 10.
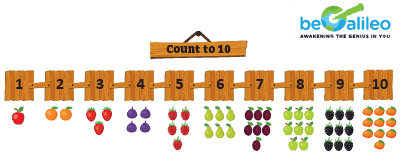
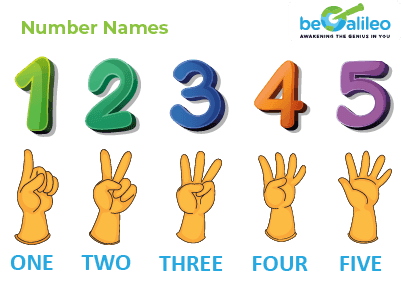
Number Names
Number names are the words used to represent numbers in written form. Each number has a specific word associated with it. For example, the number 5 is represented as "five" in words. Number names are important for reading and writing numbers and understanding their place value.
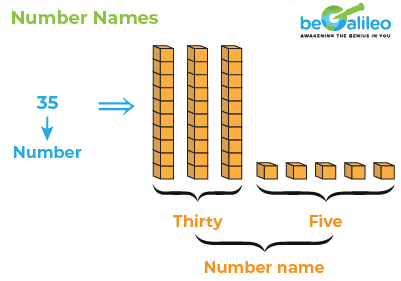
Example: The number 35 is written as "thirty-five" in number names
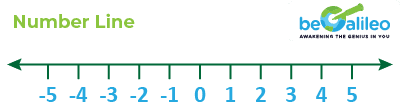
Number Line
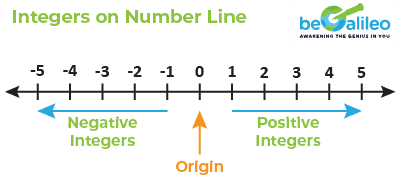
A number line is a visual representation of numbers arranged in order on a straight line. It helps us understand the relative positions and magnitudes of different numbers. The number line extends infinitely in both directions, with zero at the center. Positive numbers are represented to the right of zero, while negative numbers are represented to the left.
Example: Consider a number line with the range -5 to 5. The numbers are positioned as follows:
-5 -4 -3 -2 -1 0 1 2 3 4 5
Comparing and Ordering Numbers
Comparing and ordering numbers is the process of arranging numbers in increasing or decreasing order based on their values. It helps us understand the relative sizes and magnitudes of different numbers. For example, when comparing the numbers 3 and 7, we can determine that 7 is greater than 3.
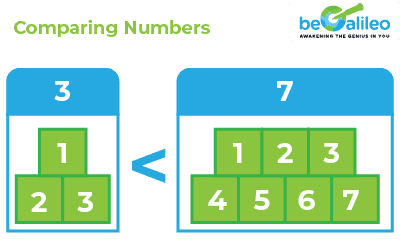
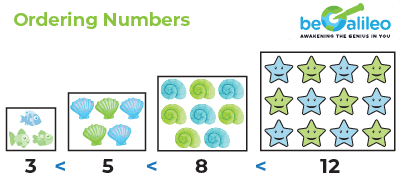
Example: Order the numbers 8, 3, 12, and 5 in ascending order: 3, 5, 8, 12.
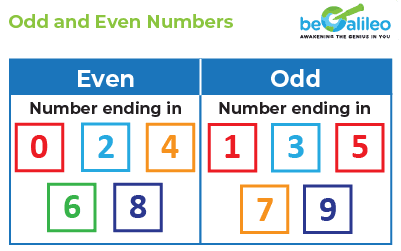
Odd and Even Numbers
Numbers can be classified as odd or even based on their divisibility by 2. Even numbers are divisible by 2, while odd numbers are not. For example, 4 and 10 are even numbers, while 5 and 11 are odd numbers. Understanding odd and even numbers helps in various mathematical operations and patterns.
Example: Identify whether the numbers 7, 12, 15, and 20 are odd or even: 7 (odd), 12 (even), 15 (odd), 20 (even).
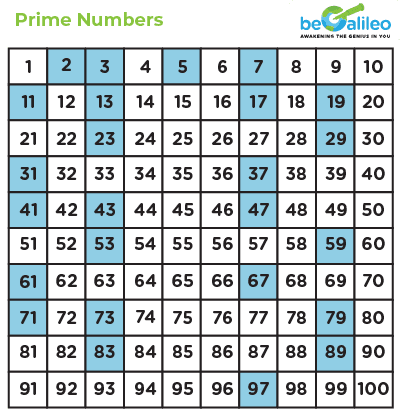
Prime Numbers
Prime numbers are numbers greater than 1 that have exactly two factors: 1 and the number itself. In other words, they are not divisible by any other number except 1 and themselves. For example, 2, 3, 5, 7, 11, and 13 are prime numbers. Prime numbers have fascinating properties and play a crucial role in number theory and cryptography.
Example: Identify the prime numbers between 1 and 20: 2, 3, 5, 7, 11, 13, 17, 19.
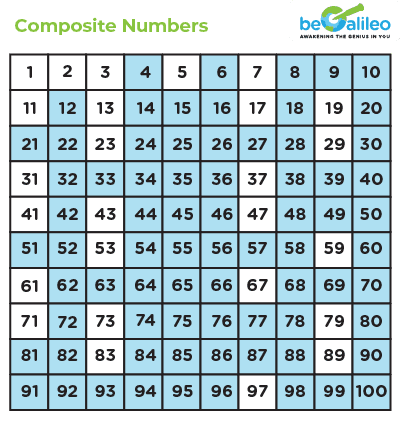
Composite Numbers
Composite numbers are numbers that have more than two factors. In other words, they are divisible by numbers other than 1 and themselves. For example, 4, 6, 8, 9, and 10 are composite numbers. Understanding composite numbers helps in identifying patterns and relationships between numbers.
Example: Identify the composite numbers between 1 and 20: 4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20.
Ordinal Numbers
Ordinal numbers represent the position or order of objects in a sequence. They are derived from counting numbers and indicate the rank or place of an object in a series. For example, first, second, third, and so on. Ordinal numbers are important for understanding rankings, ordering, and arranging objects in a specific order.

Example: Write the ordinal numbers for the counting numbers 1 to 5: first, second, third, fourth, fifth

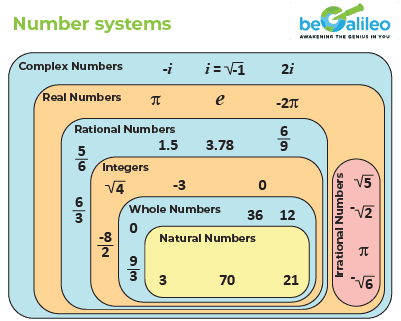
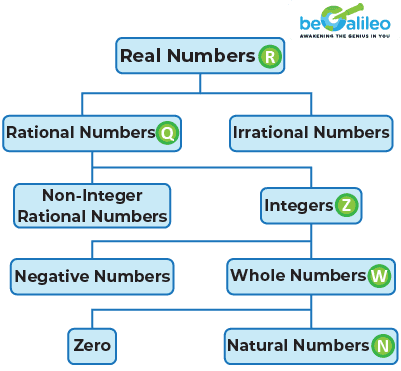
Types of Numbers
Numbers can be categorized into different types based on their properties. The numbers are
Natural Numbers
Natural numbers are the counting numbers that start from 1 and continue indefinitely. They are denoted by N = {1, 2, 3, 4, 5, ...}.
Example: 1, 2, 3, 4, 5, ...
Whole Numbers
Whole numbers include natural numbers along with zero. They are denoted by W = {0, 1, 2, 3, 4, ...}.
Example: 0, 1, 2, 3, 4, ...

Integers
Integers are numbers that include both positive and negative whole numbers along with zero. They are denoted by Z = {..., -3, -2, -1, 0, 1, 2, 3, ...}.
Example: ..., -3, -2, -1, 0, 1, 2, 3, ...
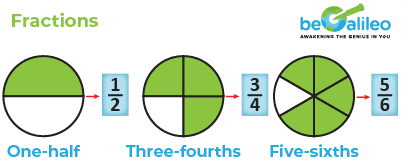
Fractions
<a href=\"/math/numbers/fractions\">Fractions</a> represent a part of a whole or a ratio between two numbers. They are expressed as , where a is the numerator and b is the denominator. For example, , and are fractions.
Example: , , , ...
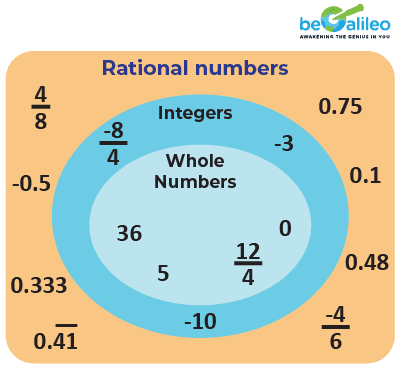
Rational Numbers
Rational numbers include integers and fractions. They can be expressed as the ratio of two integers. Rational numbers can be positive, negative, or zero. For example, , , and 4 are rational numbers.
Example: , , 4, ...
Irrational Numbers
Irrational numbers cannot be expressed as fractions or ratios. They are non-repeating and non-terminating decimals. Examples of irrational numbers include , π (pi), and e (Euler's number).
Example: , π, e, ...
Real Numbers
Real numbers include both rational and irrational numbers. They represent all possible numbers found in the real world. Real numbers can be positive, negative, or zero. They are denoted by the symbol R.
Example: -∞, ..., -3, -2, -1, 0, 1, 2, 3, ..., ∞
Complex Numbers
Complex numbers consist of a real part and an imaginary part. They are represented in the form a + bi, where a and b are real numbers, and i is the imaginary unit (). Complex numbers are used in advanced mathematics and have applications in fields like engineering and physics.
Example: 2 + 3i, -4 + 5i, ...
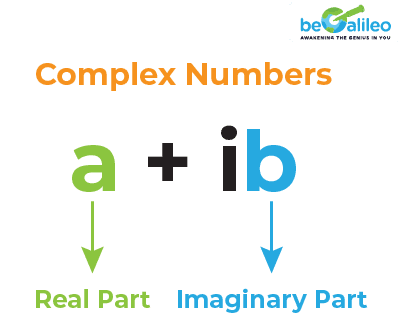
Interesting Facts about Numbers
- The number zero (0) is neither positive nor negative and is considered an important mathematical concept.
- The number 1 is unique as it is not classified as a prime number or a composite number.
- The number pi (π) is an irrational number that represents the ratio of a circle's circumference to its diameter. It is approximately equal to 3.14159 and is used in various mathematical calculations.
- The Fibonacci sequence is a series of numbers in which each number is the sum of the two preceding ones. It has intriguing mathematical properties and can be found in various natural phenomena. For example: 1, 1, 2, 3, 5, 8 ...
- The number 7 is often considered lucky in many cultures, while the number 13 is associated with superstitions and considered unlucky in some traditions.
Solved examples
Q1. Which of the following numbers is an integer?
- a)
- b)
- c)
- d)
Solution: The correct answer is .
As
Q2. Identify the rational number.
- a)
- b)
- c)
- d)
Solution: The correct answer is
is the only rational number as the recurring decimal can be written as a fraction,
Q3. Identify the natural numbers among the given numbers:
- a)
- b)
- c)
- d)
- f)
- g)
Solution: Since negative numbers, 0, decimals, and fractions are not a part of natural numbers. Therefore, 0, -98, and are not natural numbers.
Therefore, natural numbers are 23, 98, and 3.
Q4. Are all the fractions rational numbers?
- a) Yes
- b) No
Solution: Fractions are numbers that are represented in the form of (numerator/denominator), which is equivalent to form, so fractions are considered rational numbers.
Example: is a fraction and it is also a rational number.
Q5. Are all rational numbers fractions?
- a) Yes
- b) No
Solution: All fractions are rational numbers, but all rational numbers are not fractions
Practice Problems
1. Is a rational number?
Yes
No
2. Is a fraction?
Yes
No
3. Ron says all rational numbers are fractions and Sara says all the fractions are rational numbers. Who is correct?
Ron
Sara
Both of Them
None of Them
4. Choose a number that is not rational.
-200
5. is an integer. Given that the value of m lies between 29 and 39. Choose the value of m.
m = 24
m = 36
m = 38
m = 30
Frequently Asked Questions
What age group is beGalileo designed for?
Can I try beGalileo before committing to a package?
What is the difference between self-paced and live class options in beGalileo?
How can beGalileo support homeschooling parents?
What curriculum does beGalileo follow?
How can I track my child's progress?
What is the frequency and duration of beGalileo tutoring classes?
Can beGalileo's program be customized for my child's specific learning requirements?
Can your teachers teach topics covered in my child's school curriculum?
Can my child join beGalileo anytime during the year?
What if I am not satisfied with beGalileo's classes after enrolling?
How do I enroll for beGalileo's classes?



Did you know?
The number zero (0) is neither positive nor negative and is considered an important mathematical concept.














